Modal expansion
- The nature world is continuous
- Once measured, a signal is discrete.
- Volt versus time
- Elevation map
- Image
- Chose the modal basis adapted to your problem.
- Goal is to try to reduce the number of parameter to describe function, and make use of convenient properties of this description
- Examples:
- An optical phase using Zernike modes
- Finite Element Model analysis
- Eigenvalues engenmodes
- Cyclic signals are naturally described by expanding on sines and cosines
The Fourier Transform
What is the Fourier Transform?
- The Fourier transform of a signal tells you what frequencies are present in your signal and in what proportions


- IMHO, the most useful mathematical tool for engineers and applied physicists.
- It is used to:
- Characterise signals (1D/2D..) and linear systems. Electronics, optics, acoustics, mechanics, civil engineering, etc, etc…
- Digitally process data/signals (filtering, convolving, correlating, etc) in all above disciplines
Fourier Filtering


Fourier transform: definitions
- We note the Fourier transform of
- The inverse Fourier transform is
- We will also use the Fourier operator :
- The Fourier transform is cyclic:
- To have a Fourier transform, a function must
- Be absolutely integrable
- Not have any infinite discontinuity
- Have only a finite number of discontinuities or extrema in any finite interval
Fourier Pairs
| Function | Fourier Pair |
|---|---|
Properties
| Property | Expression |
|---|---|
| Linearity | if |
| Similarity | |
| Shift | |
| Convolution | |
| Autocorrel. | |
| Parseval | |
| Power | |
| Derivative |
2D FT, DFT, FFT, PSD
- Acronyms:
- FT: Fourier Transform
- DFT: Discrete FT
- FFT: Fast FT
- PSD: Power Spectral Density (modulus square)
2D Fourier transform
The Forward transform is:
And the Reverse is
- Note that if can be factorised (convenient) then
- (but if the problem is more complicated ...)
- All other theorems apply as in 1D (linearity, similarity, power, etc)
Some 2D Fourier pairs


Discrete FT and Fast FT
- The Fourier transform can be modified for discrete datasets, which is extremely useful to represent and analyse sampled physical signals. The discrete Fourier transform (DFT) is:
- and are discrete variables. Both functions consist of sequences of N samples. Basic theorems for the FT also apply to the DFT.
- The Fast Fourier Transform (FFT) is a DFT that uses a smart algorithm to drastically reduce the number of operations, from down to
The Power Spectral Density (PSD)
- The square modulus of the Fourier transform of a signal
- PSD is insensitive to the phase of the input signal.
- you get the power (intensity) per frequency bin over the frequency range 0 to cut off frequency
- In a DFT, assuming:
- the units of x are seconds (s),
- and the units of f, say, Volts (V)
- then the PSD units are V/Hz.
See Spectrum Density Analyser
Diffraction Theory
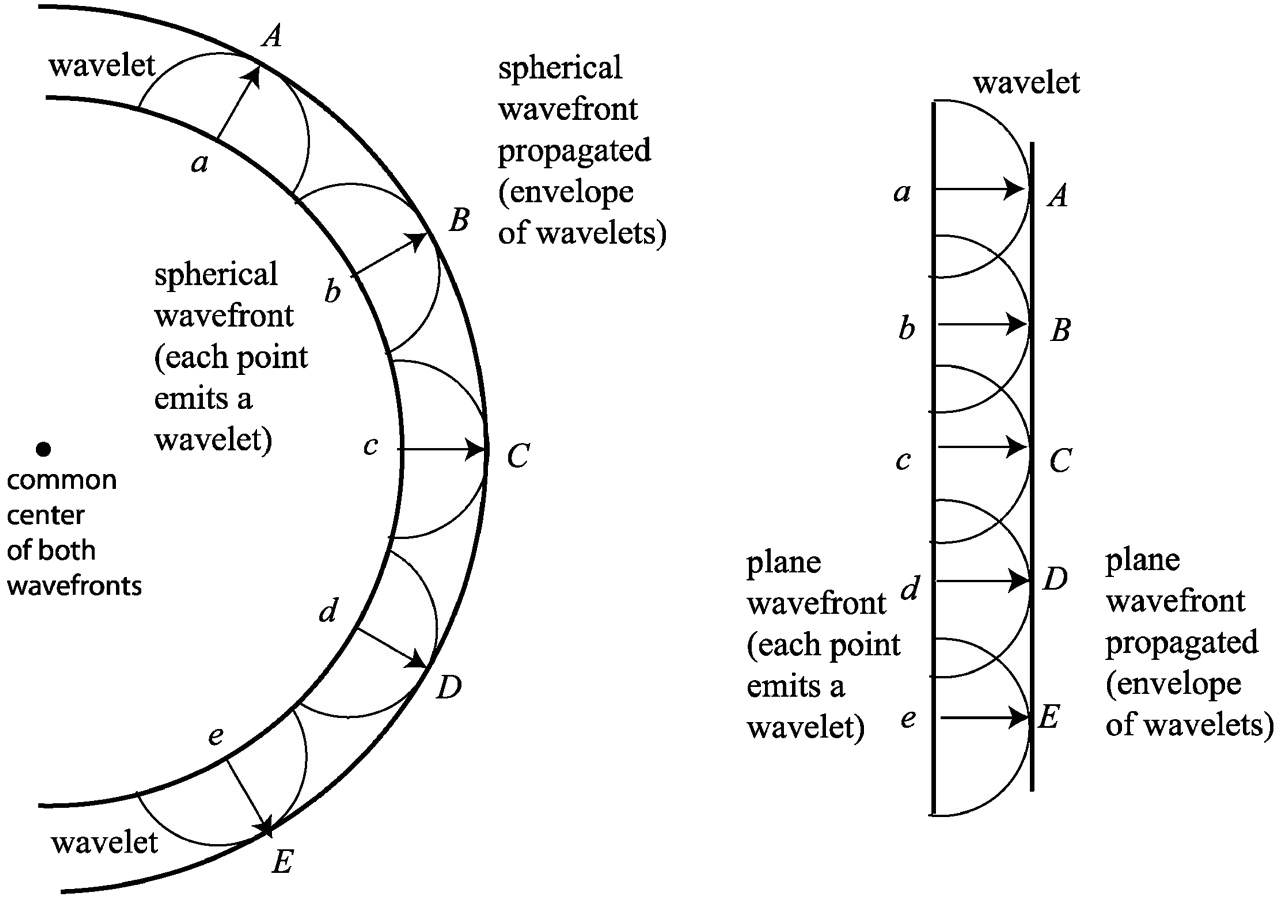
Huygens Principle
- "Every point on a wavefront may be considered a source of secondary spherical wavelets which spread out in the forward direction at the speed of light. The new wavefront is the tangential surface to all of these secondary wavelets."
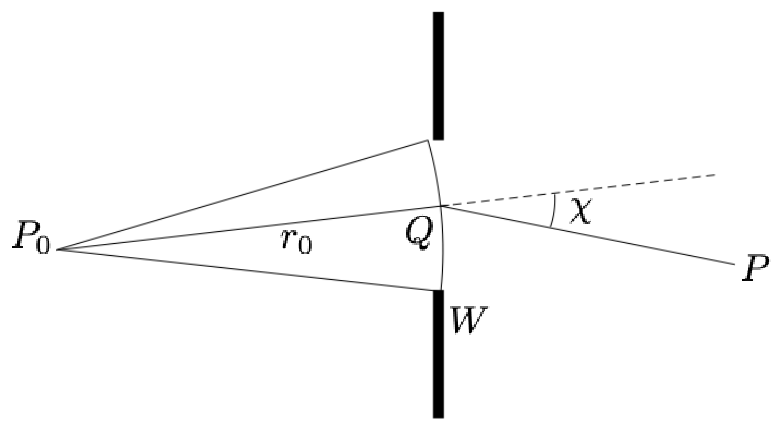
(Non) Derivation of the diffracted field
 |
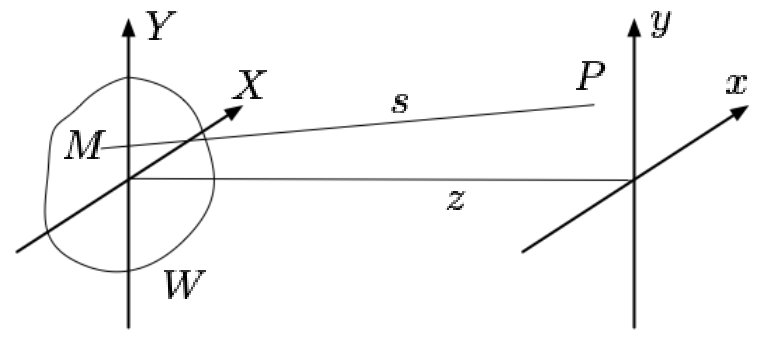 |
- Fresnel, KrichHoff and Sommerfeld, within others, have worked out the math. It's messy, and requires a lot of approximations.
- Applying the Huygens principle and working out the field propagation from the point through the aperture in plan (near field), to the final plan (far field), it can be demonstrated that the field in is the simple Fourier Transform of the field in :
Wavefront, PSF, OTF
The Impulse Function
- Recalling the field in P:
- At visible wavelengths, it is extremely difficult to measure the complex field itself (for quantum noise reasons) - but we can measure the field intensity (irradiance), the square of the complex field. H is the image of a point, the impulse function, also called the Point Spread Function (PSF):
Remember that ? So, in absence of aberrations (), we simply have:
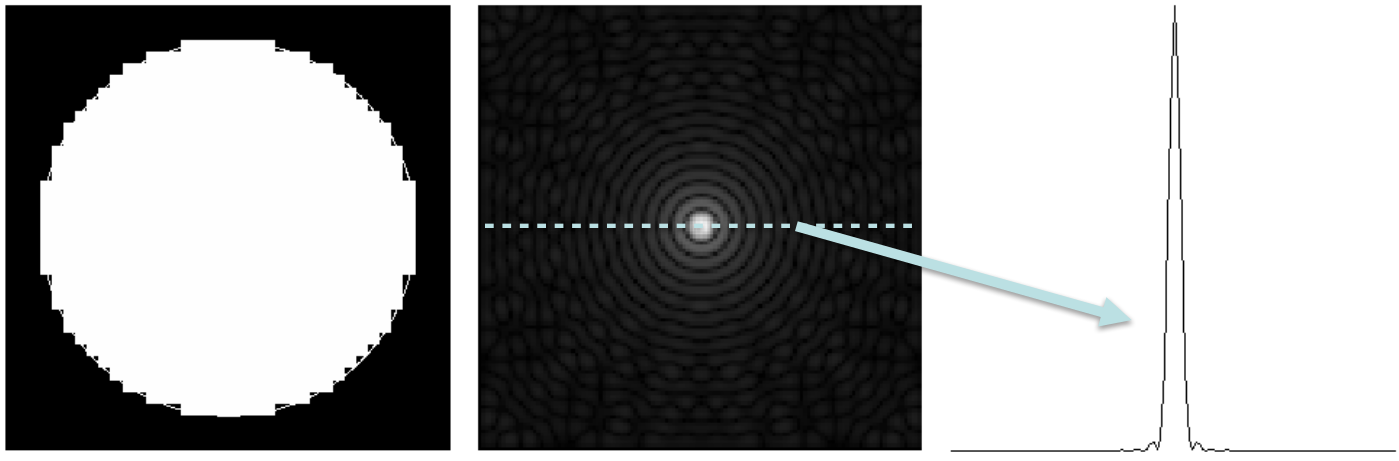
The Impulse Function, circular aperture
For a circular aperture:

An Application: Young Fringes
-
Armed with this new mathematical description of diffraction, it is now trivial to find, e.g., the expression of the Young fringes.
-
The
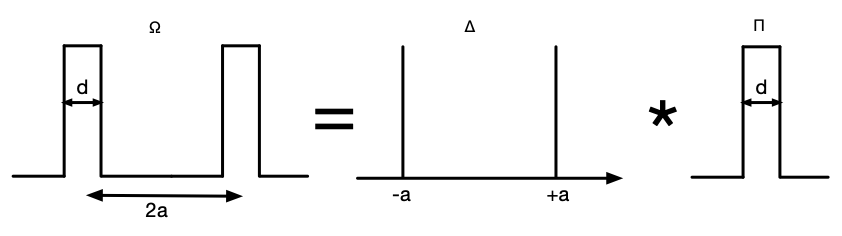 slits can be described as a convolution:
slits can be described as a convolution: -
The near field can be written
-
The far field is
-
hence
-
and hence
Thus and the intensity (measured)
Airy pattern, circular aperture PSF
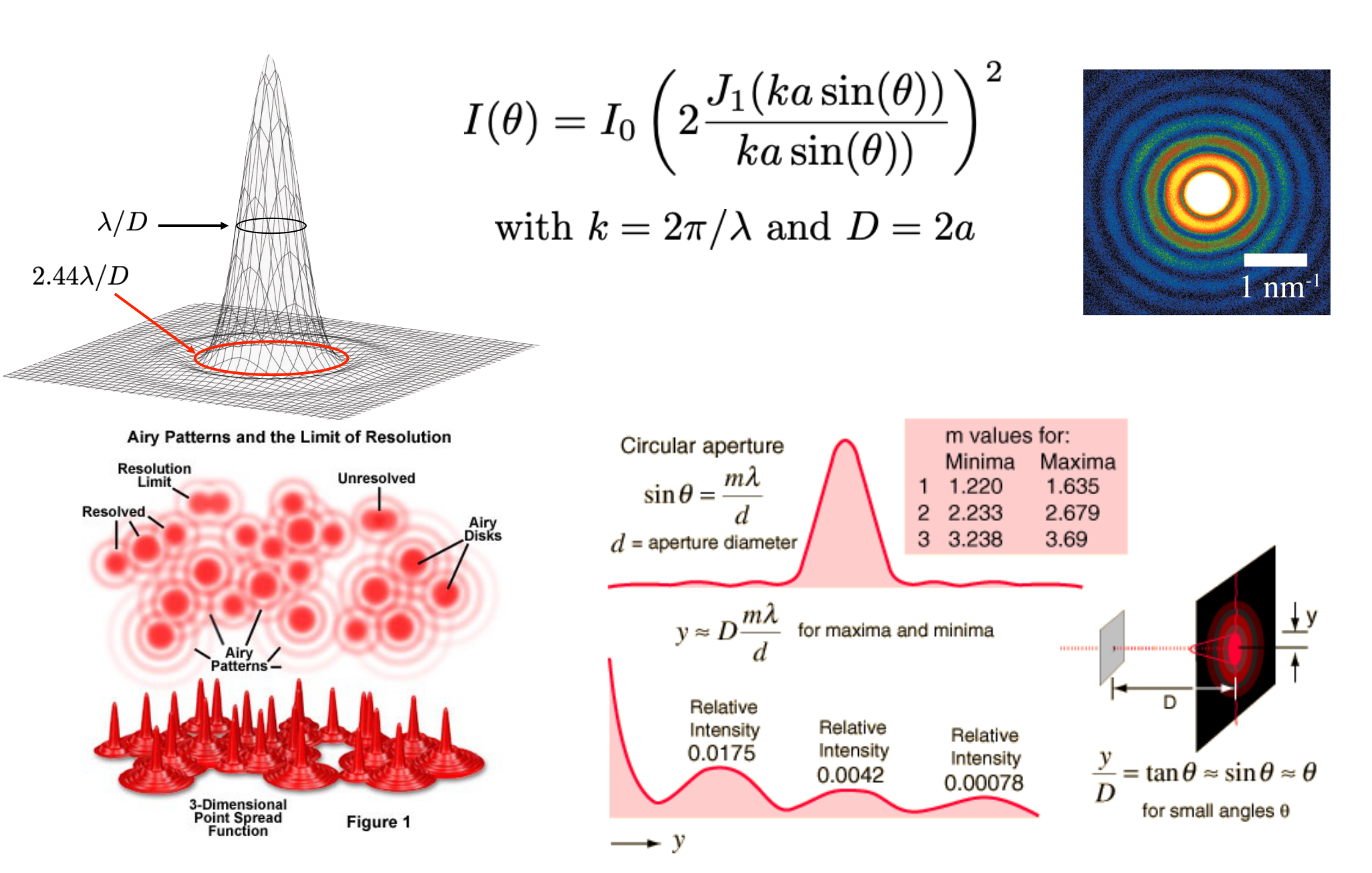
The Optical Transfer Function
- Also called generically Modulation Transfer Function (MTF)
- Characterises the filtering properties of an optical system, including cut-off frequency
- For a circular aperture, the cut-off frequency is
- People have written books about it…
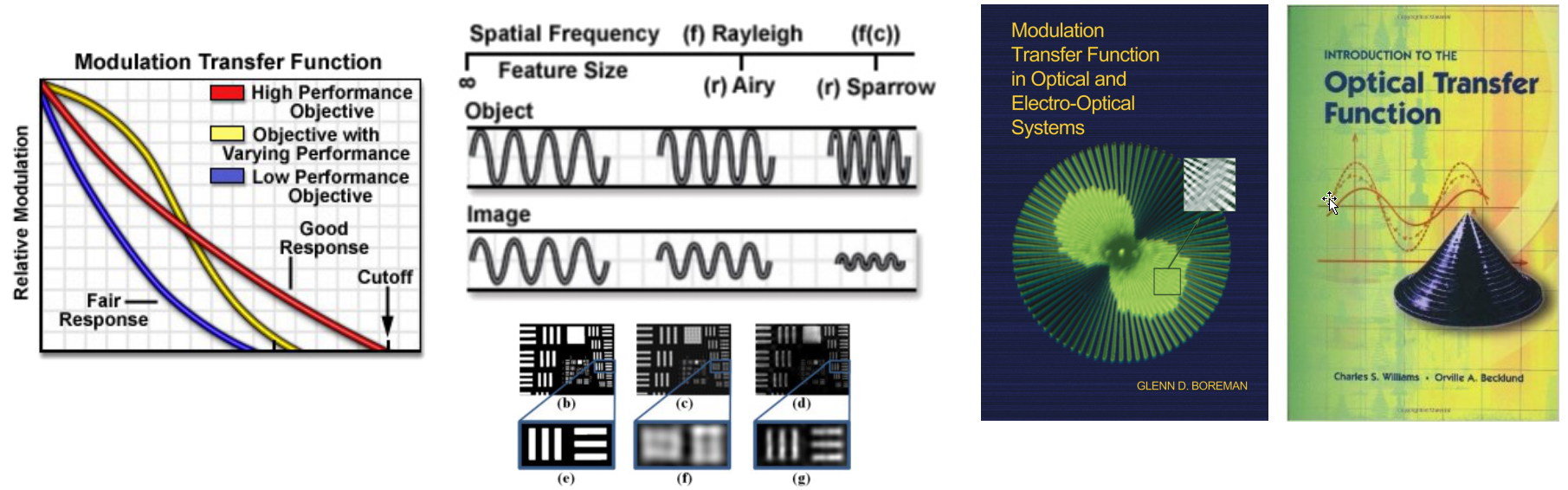
Wavefront, PSF & OTF are linked
- The wavefront is
- is the complex field defined by its amplitude and phase
- is the amplitude (e.g. pupil function)
- is the phase
- The Optical Transfer Function (or MTF) is the spatial frequency response of the system.
- Wavefront, PSF and OTF are linked:
Interferometry to Imaging
...and Back
Image formation for incoherent sources
An  object O can be decomposed into an infinite number of dirac function. In the case of an incoherent object (most objects in everyday’s life, astronomical objects, medicine,etc), these points do not interfere, thus the resulting image is the convolution of the object and the impulse response (PSF)
object O can be decomposed into an infinite number of dirac function. In the case of an incoherent object (most objects in everyday’s life, astronomical objects, medicine,etc), these points do not interfere, thus the resulting image is the convolution of the object and the impulse response (PSF)

Note that this assumes invariance of PSF with position in the field of view.
Interferometry to Imaging...
From "slit" to full aperture
= Aperture
= Pupil
= Focal plane Image
Interferometry to Imaging...
From "slit" to full aperture

Interferometry to Imaging...
From "slit" to full aperture

Interferometry to Imaging...
From "slit" to full aperture

Interferometry to Imaging...
From "slit" to full aperture

Interferometry to Imaging...
From "slit" to full aperture

Interferometry to Imaging...
From "slit" to full aperture
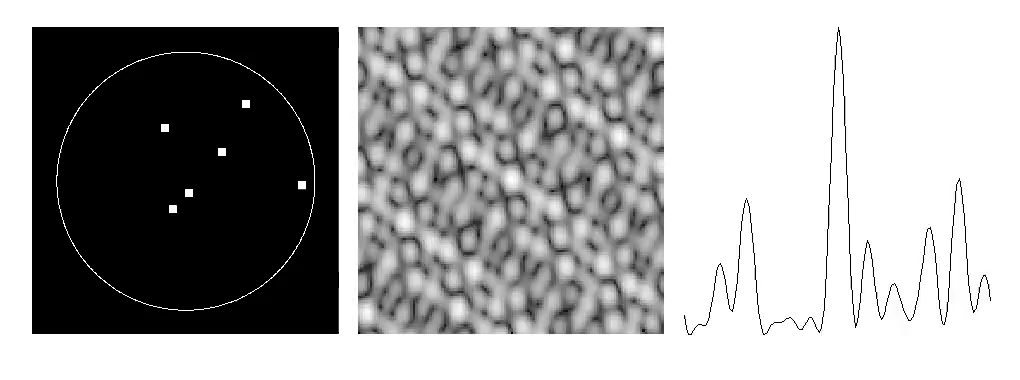
Interferometry to Imaging...
From "slit" to full aperture
... and Imaging to Interferometry
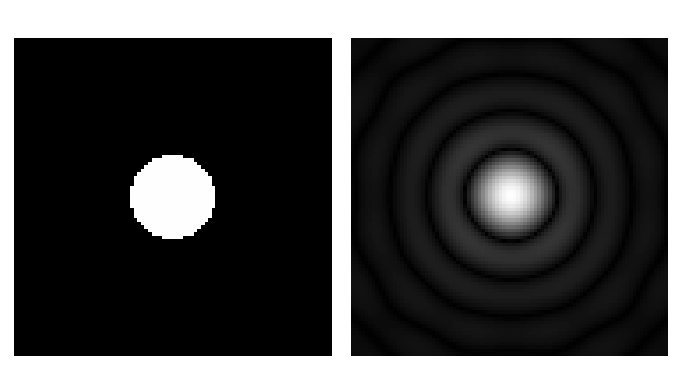
... and Imaging to Interferometry
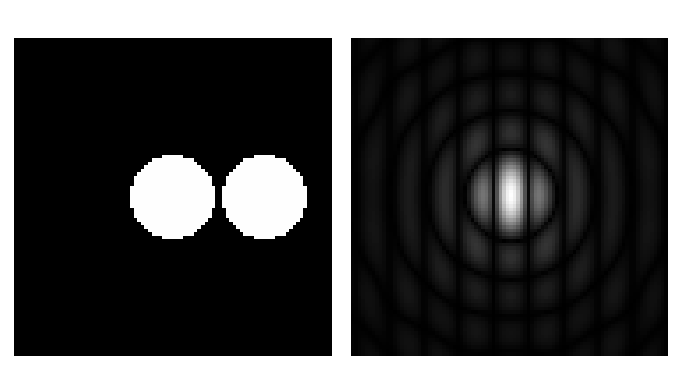
... and Imaging to Interferometry

... and Imaging to Interferometry
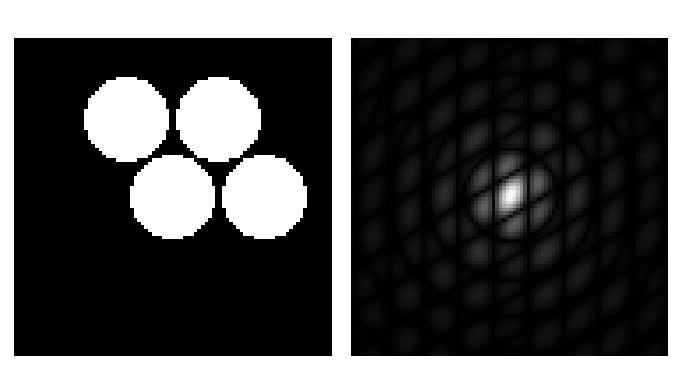
... and Imaging to Interferometry

... and Imaging to Interferometry
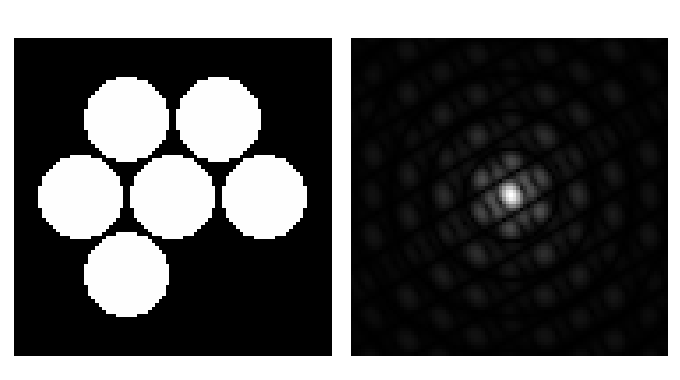
... and Imaging to Interferometry

Elements of Sampling Theory
Sampling & Aliasing (Shannon/Nyquist)
If a continuous, band-limited function contains no frequency component higher than , then it can be fully specified by a set of samples at frequency of or larger.
Sampling and the Shah function
is also called a frequency comb.
-
is its own Fourier transform
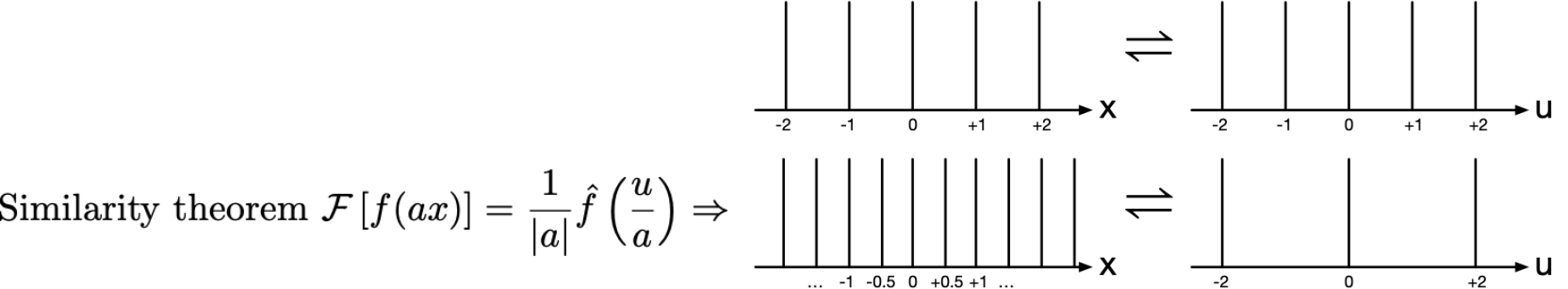
-
The act of sampling is taking value at discrete points Multiplication by

Aliasing
- Can be spatial, temporal, angular, etc

- Can be solved/mitigated by pre-filtering the signal before sampling

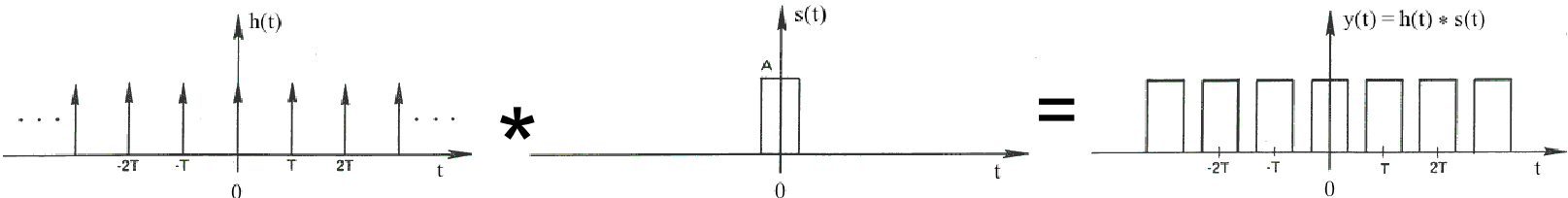
The Shah function (part 2)
Convolution by is equivalent to creating an infinite number of shifted replicas of the original functions
Aliasing
Fourier view: In the DFT/FFT space, the function spectrum is replicated at intervals . If the spectrum spills over , then the spectrum replicas will overlap, resulting in a mixed signal (original lost).

proof of the sampling theorem
From we obtain the sampled with sampling interval by:
In the Fourier domain:
The spectrum of the sampled function consists of an infinite sum of replicas of . If , the replicas are separated by distances larger than their width and do not overlap (if not, they do and it creates in aliasing). Hence the information on and thus on is preserved if the sampling condition is met. We can retrieve the original spectrum by multiplying by a rectangle function (gate) in order to eliminate all replicas but one:
which yields by inverse Fourier transform
Main points of past two lectures
-
Basic understanding of the Fourier transform and its properties, sampling and aliasing issues
-
In Fourier Optics, light is described by a scalar field
-
In Fraunhofer diffraction, the far and near field complex amplitudes are linked by a Fourier transform
-
An optical system can be characterised by its impulse function . The impulse function is
-
Object and image are linked by the relation
-
The Optical Transfer Function of a system characterises its spatial frequencies filtering properties
Image Metrics, Aberations
Practical Optical Systems

Image metric: Full-Width at Half-Maximum
- The width of the image at half its maximum. Often written FWHM
- For instance, a cross section of a gaussian image
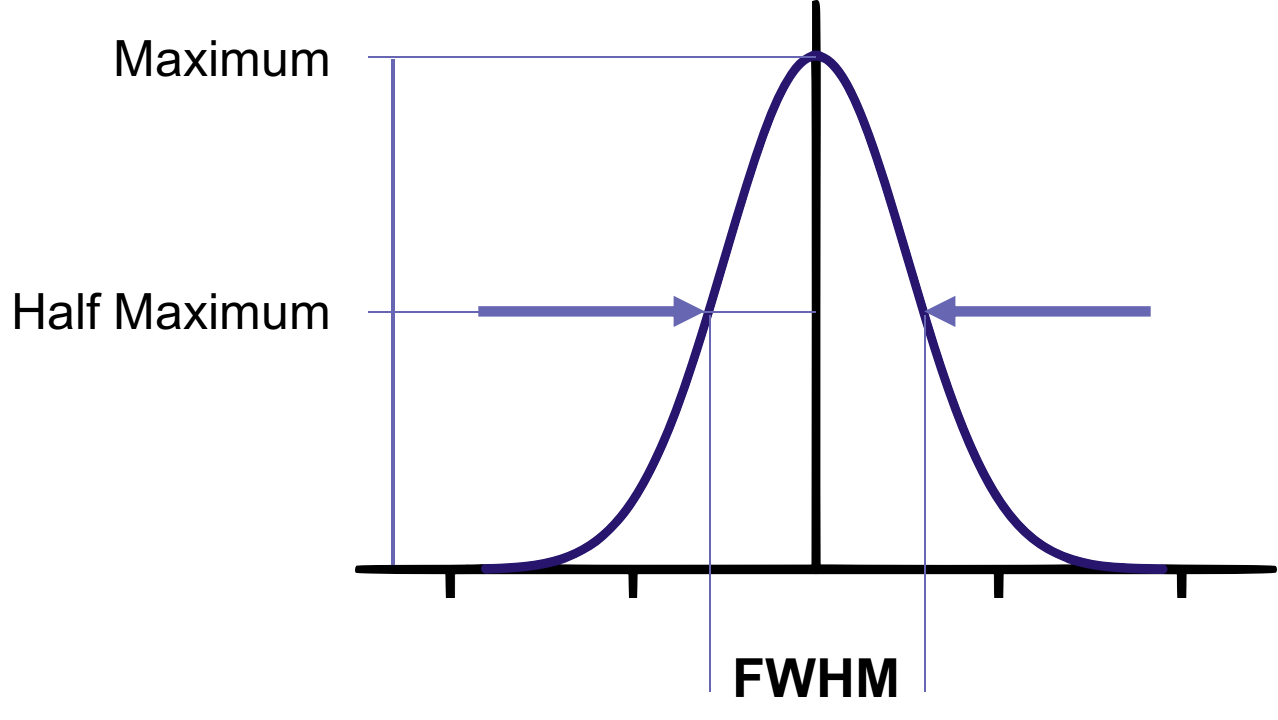
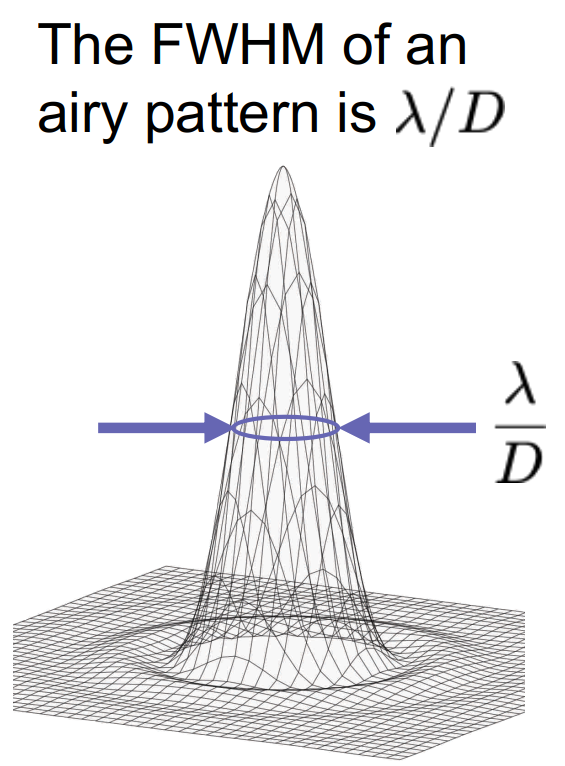
- The FWHM is often naturally expressed as an angle (e.g. arcsec) or a distance (e.g. mm), as it often characterise a resolution
Image metric: Strehl ratio
- The ratio between the maximum intensity in the actual image to the maximum in a diffraction limited image.
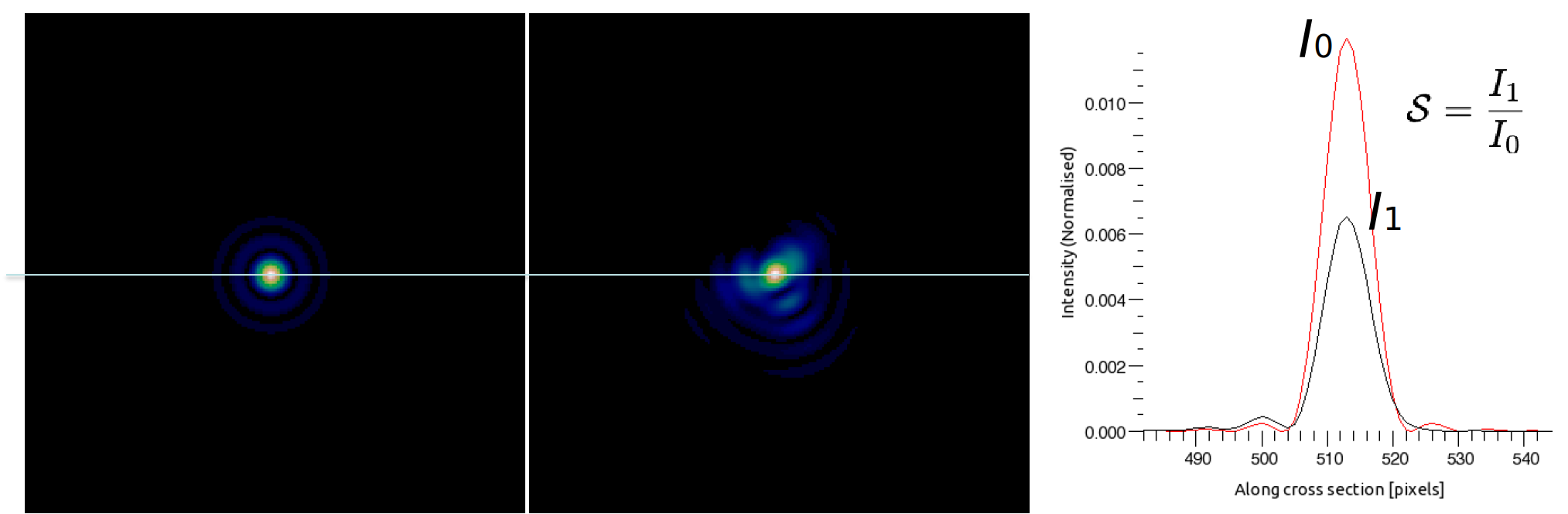
- A measure of how much energy is in the diffraction limited core
- For
- . The Strehl ratio is often expressed in % (%)
Image metric: Encircled Energy
- Intensity within a certain radius normalised by total intensity of the image

Beyond "Simple" Metrics
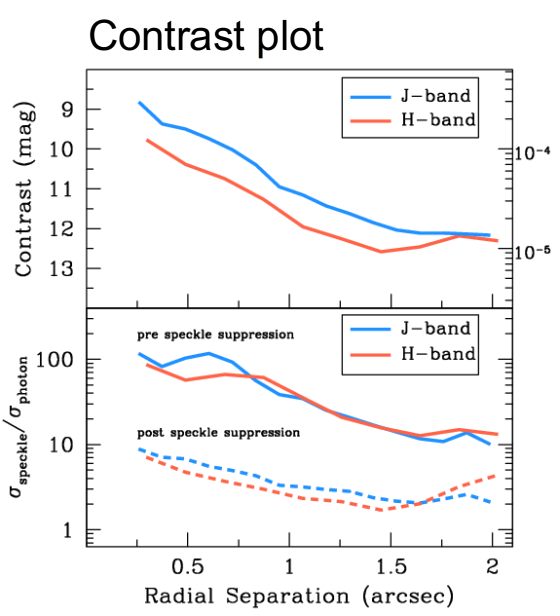
- HighHD8375. J.Crepp et al 2013contrast imaging required the development of new metrics and new techniques to improve contrast performance
 HR8799. T.Currie et al 2012
HR8799. T.Currie et al 2012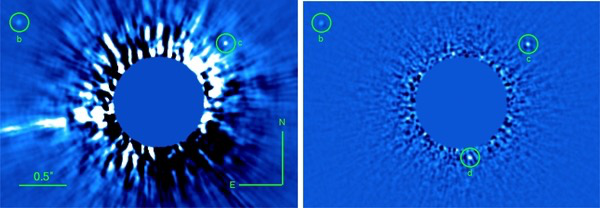
- Speckle control